
Sundial Park Genk
8. Digital sundial

Be it accidental or not, the four most innovative sundials line the high side of the Sundial Park: the digital dial, the conical dial (nr. 9) and the shadow plane dials (nr. 10 and nr. 11).
"What in heaven is a digital sundial?" That was the title of an article in Scientific American (August 1991), which prompted a flurry of mental activity in many a smart brain. One answer is on display here: a sundial that displays the time, not by throwing a shadow on a set of lines, but in plainly readable digits. The dial functions from 8:00 to 5.55 hr, in 5 minute steps. There is a smooth transition from one reading to the next. In the picture above, the "0" from 3:20 is still faintly visible.
The sundial has been invented by Hans Scharstein and Werner Krotz-Vogel (Germany) and Daniel Scharstein (USA). See for more information the website of Digital Sundials International.
My comments:
A real black box!
 When we first visited the Sundial Park, I saw this shallow black box high on a pole, still needing support apparently. The weather was heavily overcast, and the object gave no indication of any sundial functionality, be it that the short rod carrying the box seemed to be pole-stylish. It looked as if something could be displayed on the black screen, but no electrical connection could be seen. Sine sole sileo (I am silent without sun) would be a most adequate motto for this device!
When we first visited the Sundial Park, I saw this shallow black box high on a pole, still needing support apparently. The weather was heavily overcast, and the object gave no indication of any sundial functionality, be it that the short rod carrying the box seemed to be pole-stylish. It looked as if something could be displayed on the black screen, but no electrical connection could be seen. Sine sole sileo (I am silent without sun) would be a most adequate motto for this device!
Principle of the digital dial
The sundial consists of two closely spaced parallel masks. The first mask, facing the sun, has a regular array of thin vertical slits, which cast a striped light pattern onto the second mask. That mask, facing the visitor, is composed of narrow stripes, cut from the digits to be displayed. These stripes are intermingled, so that they are illuminated consecutively. The figures below illustrate this in a simplified example for the right-most numeral, which is either a "0" or a "5".
mask facing sun |
mask facing visitor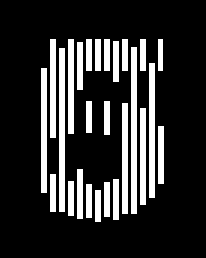 |
In one position of the sun, the stripes cut from the "0" are lit. Five minutes later the sun has moved over 1.25° and the stripes derived from the "5" are illuminated. Again 5 minures later, the stripes from the "0" are again illuminated, but the light falling through a given slit has advanced one stripe to the left.
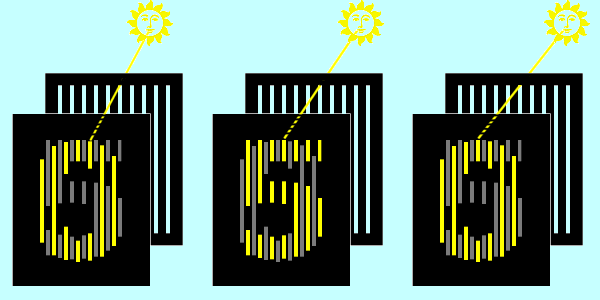
A translucent screen behind the second mask helps diffusing the thin light lines and enables the numerals to be read from a broad angle. Narrow your eyes, if necessary, to simulate this effect.
 In reality the twelve numbers for the minutes display: 00, 05, 10, ..., 50, 55, are cut and intermingled together. So only after one hour will the light falling through a given slit has advanced one stripe to the left. Similarly, the ten hour numbers: 8, ..., 12, 1, ..., 5 are mixed in the left half of the box. The fineness of the fabrication process is obvious from the picture at right, showing a detail from the top one: between each pair of light lines, eleven others will appear successively in one hour time.
In reality the twelve numbers for the minutes display: 00, 05, 10, ..., 50, 55, are cut and intermingled together. So only after one hour will the light falling through a given slit has advanced one stripe to the left. Similarly, the ten hour numbers: 8, ..., 12, 1, ..., 5 are mixed in the left half of the box. The fineness of the fabrication process is obvious from the picture at right, showing a detail from the top one: between each pair of light lines, eleven others will appear successively in one hour time.
The slits and the stripes are pointing to the celestial pole, therefore the date (declination of the sun) does not play a role. The principle and construction of the digital dial is described in more detail in the patent application.
The trick that makes it really work
That sounds not too complicated, isn't it? No, but it wouldn't work very well this way...
In fact, each slit in the first mask is the gnomon of a small polar dial. The stripes in the second mask are the time lines: 5-min lines for the minute numbers, 1-hour lines for the hour numbers.
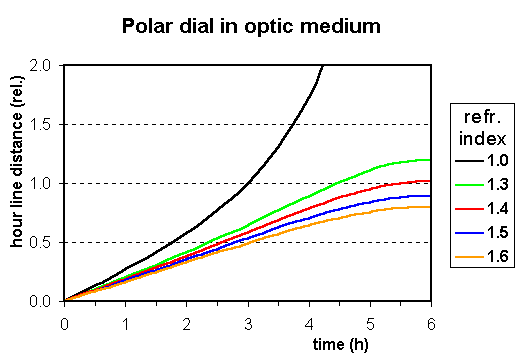
First, have a look at the dial face of a polar dial, such as the Book of Time (nr. 12). The hour lines are not equally spaced, but their interval increases considerably to the sides. The shadow of the pole-style moves slowest across the dial face around noon, and faster in the morning and the afternoon.
Now have a look at the right-most panel in the figure above, the one with the three sun icons. Assume that we are here somewhere in the afternoon. The effect of increasing velocity of the lines of light would cause them to hit the next stripes just somewhat too early. The effect would get stronger as the sun moves further to the west, so that the time would advance at an increasing pace. And that is not what a clock is expected to do...
What is needed is a way of linearizing the relationship between solar angle (corresponding to time) and the velocity of the light across the second mask.
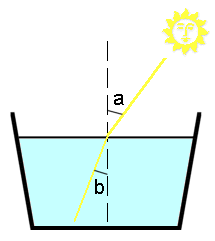 This problem can be solved by profiting from an oddity of nature, and that is in my view the real heart of this invention. This 'oddity' is Snell's law of refraction, which describes how light traverses the boundary between two media. The relationship between angle a (in air) and b (in some transparent medium) depends on the index of refraction n of the medium:
This problem can be solved by profiting from an oddity of nature, and that is in my view the real heart of this invention. This 'oddity' is Snell's law of refraction, which describes how light traverses the boundary between two media. The relationship between angle a (in air) and b (in some transparent medium) depends on the index of refraction n of the medium:
sin(b) = sin(a)/n .
Four large digital dials installed
The digital dial in Genk was the first one on public display world-wide. Next, the Sundial Garden in the Deutsches Museum in Munich (Germany) had one installed, followed by the Municipal Museum in Köln (Cologne, Germany). The latest one is in front of a house on Martha's Vineyard, an island off the coast of Massachusetts (USA).
The window-sill version
A smaller version of the digital dial has been developed that can be placed on the window-sill of a south-facing window. The minutes now are displayed in a column to the right of the hour numbers, in 10 min steps. The advantage is that the transition from one number to the next does not occur at the same place. This enables accurate reading, by interpolating the observed intensities. In the example below, it is between 12:35 and 12:40 hr.

A new type, or just a multiple polar dial?
 Is this just a multiple polar dial, comparable to multiple dials like the polyhedron dial (nr. 4)? Some people think so. In my view, that does not do justice to the degree of organization of this instrument. Using an array of hundreds closely spaced, interacting polar dials to get
pictures out of the instrument is quite an innovative step. As a metaphor, compare a single string under tension that produces a single tone when hit with a piano on which a
Beethoven sonata could be played (or a boogie-woogie if one preferred).
Is this just a multiple polar dial, comparable to multiple dials like the polyhedron dial (nr. 4)? Some people think so. In my view, that does not do justice to the degree of organization of this instrument. Using an array of hundreds closely spaced, interacting polar dials to get
pictures out of the instrument is quite an innovative step. As a metaphor, compare a single string under tension that produces a single tone when hit with a piano on which a
Beethoven sonata could be played (or a boogie-woogie if one preferred).
Other 'digital' dials
Several sundial designers have called their product 'digital'. Examples are:
 The Thew sundial, in which an equatorial band has holes in the form of the hour numbers. These are projected onto the dial face, on which a vertical, pole-oriented line marks the time. See for instance the Whitehall dial in our garden.
The Thew sundial, in which an equatorial band has holes in the form of the hour numbers. These are projected onto the dial face, on which a vertical, pole-oriented line marks the time. See for instance the Whitehall dial in our garden.
The digital sundial of HinesLab, Inc. (USA). This is essentially (a blueprint package for) an equatorial dial, where the light is picked up by optical fibers and routed to a 7-segment digital display. No electronics involved. The display resolution is ten minutes.
Robert L. Kellogg (USA) developed a "compound polar dial" that is similar to the Scharstein dial. One sundial covers a time span of 4 hours, so that 3 dials are necessary to span a day. The display resolution is 10 min. His description was published in the NASS Compendium vol. 2 nr. 3 (1995), p. 4-10.
 The digital sundial by Dietrich Ahlers in Bremen (Germany; website in German), a more sophisticated version of the Thew dial, capable of reading civil time with 1-2 min accuracy.
The digital sundial by Dietrich Ahlers in Bremen (Germany; website in German), a more sophisticated version of the Thew dial, capable of reading civil time with 1-2 min accuracy.
 A sundial using digital (computer) power was the virtual vertical sundial designed by Willy Leenders (Hasselt, Belgium) for an exhibition in the Municipal Museum in his home town in the summer of 2000.
A sundial using digital (computer) power was the virtual vertical sundial designed by Willy Leenders (Hasselt, Belgium) for an exhibition in the Municipal Museum in his home town in the summer of 2000.